
课程介绍

Trefor Bazett 教授在 Cincinnati 大学任教时,制作了两套完整的的数学课程(微积分、离散数学),上传网络后备受欢迎!课程覆盖核心知识要点,并借助可视化的方式做了生动讲解,是基础学习和查漏补缺的优先选择。
Discrete Math是其中的离散数学课程,内容覆盖集合、命题逻辑、范式、概率空间、图论、树结构等,离散数学也是后续部分工科方向课程(例如 数据库)的基础,该课程形象的可视化与活泼的授课方式,让你更好地掌握相关的抽象数学知识。

课程讲师 Trefor Bazett,2016-2019年任职于 University of Cincinnati,担任助理教授,系列课程也是那时制作完成!目前任职于University of Victoria,主要爱好是数学教育,同时对代数拓扑感兴趣。
课程资料 | 下载

扫描上方图片二维码,关注公众号并回复关键字 🎯『MATH1071』,就可以获取整理完整的资料合辑啦!当然也可以点击 🎯 这里 查看更多课程的资料获取方式!
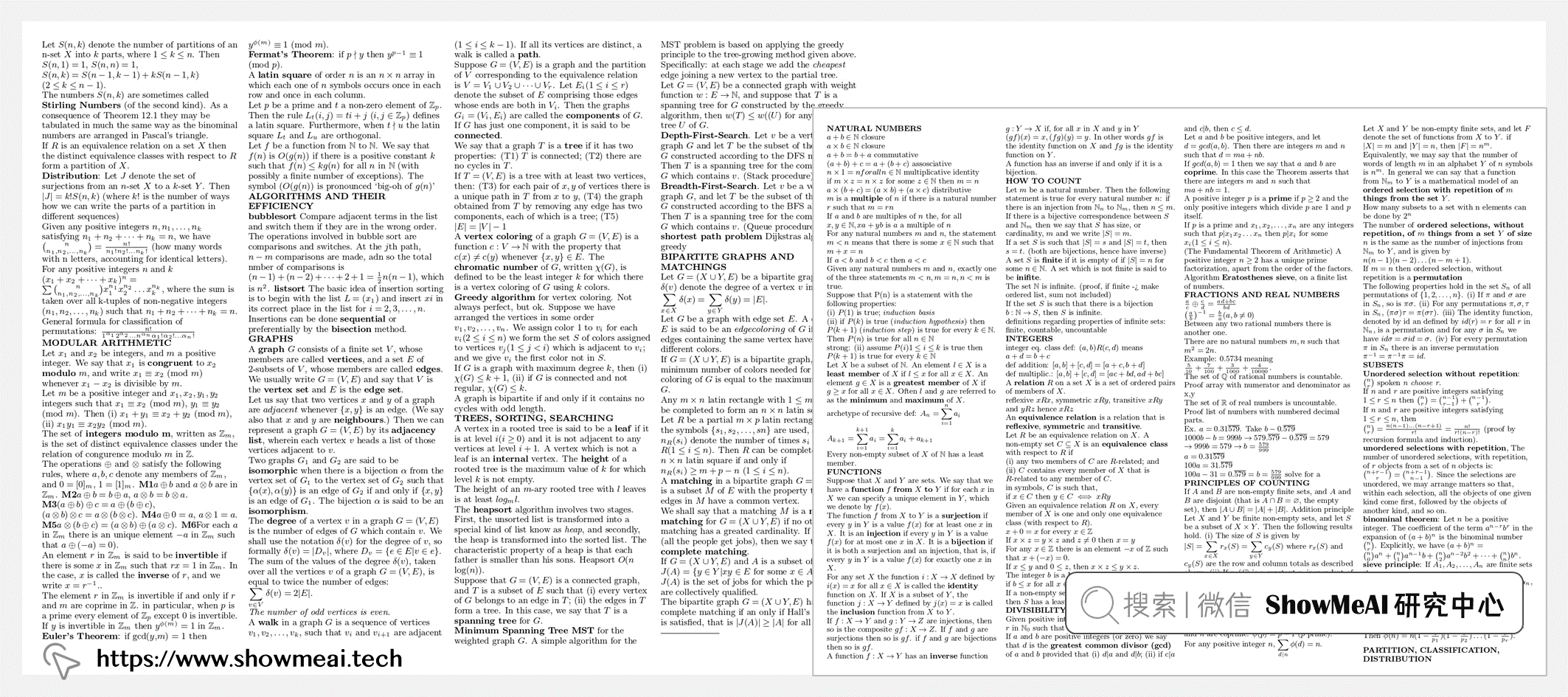
ShowMeAI 对课程资料进行了梳理,整理成这份完备且清晰的资料包:
- 📚 电子书:Mathematics for Machine Learning。
- 📚 速查表:离散数学速查表大全,将重点内容汇总整理的学习必备神器哦!
说明1:课程没有对外发布直接的课件等资料。团队搜索和筛选了能找到的、最匹配的相关资源。
课程视频 | B站
ShowMeAI 将视频上传至B站,并增加了中英双语字幕,以提供更加友好的学习体验。点击页面视频,可以进行预览。推荐前往 👆 B站 观看完整课程视频哦!
本门课程,ShowMeAI 将部分章节进行了切分,按照主题形成更短小的视频片段,便于按照标题进行更快速的检索。切分后的视频清单列写在这里:
| 序号 | 视频清单 |
|---|---|
| L1 | Intro to Discrete Math - Welcome to the Course! |
| L2 | Intro to Sets - Examples, Notation & Properties |
| L3 | Set-Roster vs Set-Builder notation |
| L4 | The Empty Set & Vacuous Truth |
| L5 | Cartesian Product of Two Sets A x B |
| L6 | Relations between two sets - Definition + First Examples |
| L7 | The intuitive idea of a function |
| L8 | Formal Definition of a Function using the Cartesian Product |
| L9 | Example: Is this relation a function? |
| L10 | Intro to Logical Statements |
| L11 | Intro to Truth Tables - Negation, Conjunction, and Disjunction |
| L12 | Truth Table Example: ~p V ~q |
| L13 | Logical Equivalence of Two Statements |
| L14 | Tautologies and Contradictions |
| L15 | 3 Ways to Show a Logical Equivalence - Ex: DeMorgan’s Laws |
| L16 | Conditional Statements: if p then q |
| L17 | Vacuously True Statements |
| L18 | Negating a Conditional Statement |
| L19 | Contrapositive of a Conditional Statement |
| L20 | The converse and inverse of a conditional statement |
| L21 | Biconditional Statements - “if and only if” |
| L22 | Logical Arguments - Modus Ponens & Modus Tollens |
| L23 | Logical Argument Forms: Generalizations, Specialization, Contradiction |
| L24 | Analyzing an argument for validity |
| L25 | Predicates and their Truth Sets |
| L26 | Universal and Existential Quantifiers, ∀ “For All” and ∃ “There Exists” |
| L27 | Negating Universal and Existential Quantifiers |
| L28 | Negating Logical Statements with Multiple Quantifiers |
| L29 | Universal Conditionals P(x) implies Q(x) |
| L30 | Necessary and Sufficient Conditions |
| L31 | Formal Definitions in Math - Ex: Even & Odd Integers |
| L32 | How to Prove Math Theorems - 1st Ex: Even + Odd = Odd |
| L33 | Step-By-Step Guide to Proofs - Ex: product of two evens is even |
| L34 | Rational Numbers - Definition + First Proof |
| L35 | Proving that divisibility is transitive |
| L36 | Disproving implications with Counterexamples |
| L37 | Proof by Division Into Cases |
| L38 | Proof by Contradiction - Method & First Example |
| L39 | Proof by Contrapositive - Method & First Example |
| L40 | Quotient-Remainder Theorem and Modular Arithmetic |
| L41 | Proof: There are infinitely many primes numbers |
| L42 | Introduction to sequences |
| L43 | The formal definition of a sequence. |
| L44 | The sum and product of finite sequences |
| L45 | Intro to Mathematical Induction |
| L46 | Induction Proofs Involving Inequalities. |
| L47 | Strong Induction |
| L48 | Recursive Sequences |
| L49 | The Miraculous Fibonacci Sequence |
| L50 | Prove A is a subset of B with the ELEMENT METHOD |
| L51 | Proving equalities of sets using the element method |
| L52 | The union of two sets |
| L53 | The Intersection of Two Sets |
| L54 | Universes and Complements in Set Theory |
| L55 | Using the Element Method to prove a Set Containment w/ Modus Tollens |
| L56 | Relations and their Inverses |
| L57 | Reflexive, Symmetric, and Transitive Relations on a Set |
| L58 | Equivalence Relations - Reflexive, Symmetric, and Transitive |
| L59 | You need to check EVERY spot for reflexivity, symmetry, and transitivity |
| L60 | Introduction to probability // Events, Sample Space, Formula, Independence |
| L61 | Example: Computing Probabilities using P(E)=N(E)/N(S) |
| L62 | What is the probability of guessing a 4 digit pin code? |
| L63 | Permutations: How many ways to rearrange the letters in a word? |
| L64 | The summation rule for disjoint unions |
| L65 | Counting formula for two intersecting sets: N(A union B)=N(A)+N(B)-N(A intersect B) |
| L66 | Counting with Triple Intersections // Example & Formula |
| L67 | Combinations Formula: Counting the number of ways to choose r items from n items. |
| L68 | How many ways are there to reorder the word MISSISSIPPI? // Choose Formula Example |
| L69 | Counting and Probability Walkthrough |
| L70 | Intro to Conditional Probability |
| L71 | Two Conditional Probability Examples (what’s the difference???) |
| L72 | Conditional Probability With Tables - Chance of an Orange M&M??? |
| L73 | Bayes’ Theorem - The Simplest Case |
| L74 | Bayes’ Theorem Example: Surprising False Positives |
| L75 | Bayes’ Theorem - Example: A disjoint union |
| L76 | Intro to Markov Chains & Transition Diagrams |
| L77 | Markov Chains & Transition Matrices |
| L78 | Intro to Linear Programming and the Simplex Method |
| L79 | Intro to Graph Theory - Definitions & Ex: 7 Bridges of Konigsberg |
| L80 | Properties in Graph Theory: Complete, Connected, Subgraph, Induced Subgraph |
| L81 | Degree of Vertices - Definition, Theorem & Example - Graph Theory |
| L82 | Euler Paths & the 7 Bridges of Konigsberg - Graph Theory |
| L83 | The End of Discrete Math - Congrats! Some final thoughts |
更多技术与课程清单 | 点击查看详细课程
